Simple tool to assess inductors and transformers with 100nH < L < 100H+
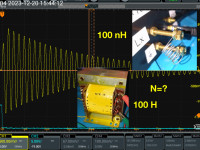
- Have an unknown inductor/transfomer? - A digital scope ..then with a tiny simple circuit and a pocket calculator you may quickly get the inductance, number of windings amount of losses using a tiny circuit in addition. Here it is described how !
A well known method to quickly get informations on the inductance L and losses of coils, transformers or other inductors is to combine them with a known capacitor to form a resonator. From the resonance frequency f one may infer the value of L. The resonance my be searched by scanning with a signal generator, using the resonator in an oscillator circuit or kicking the oscillation and observing the resulting damped oscillation. Here I will describe a tiny circuit to perform the latter method and illustrate its application with a few examples. Besides the simple "kicker" circuit (for less than 10 Euros) the method requires a digital oscillosope, which any professional and probably many hobby electronicians will have on their benches.
The method is old.
In the detailed description a very simple but versatile circuit is shown to support this method and examples are given of what can be measured and how.
This covers a small 8 turns copper coil 300 nH up to and 8000 winding transfomer core with 100 H. The only parameter to be adapted is the time base of the oscilloscope.
The description, the schematics and the examples with traces and results all are shown in the attached description.
The method is old.
In the detailed description a very simple but versatile circuit is shown to support this method and examples are given of what can be measured and how.
This covers a small 8 turns copper coil 300 nH up to and 8000 winding transfomer core with 100 H. The only parameter to be adapted is the time base of the oscilloscope.
The description, the schematics and the examples with traces and results all are shown in the attached description.
Diskussion (2 Kommentare)
A.J. Jeuring vor 7 Monaten
" with the " Inductor Tester II".
It looks like you did so. How do you mix the schematics of the "kicker"and those of the "tester II" ??
Maybe I'm wrong but still interested. Worst case scenario for me is to build both separate.
Thanks a lot. [Ik zou bij voorkeur in het Nederlands met u communiceren]
Albert Jeuring
yvon Tollens vor 1 Jahr
c'est comme cela que je faisait faire les mesures d'inductances quand j'enseignait l'electronique
dommage que certaines données soient masquées dans le pdf par le schema du circuit ,
dans les dernieres pages je ne trouve plus comment demontrer r = 2 tau / L (apres 10 ans de retraite)
je vous joint aussi : une simulation LTspice , les rappels de calculs , le tableur (odt) et l'allure d'une courbe avec les points a mesurer
Yvon Tollens
monk99 vor 1 Jahr
Concening the "masking" of some data: I cannot reproduce this, maybe an issue with the pdf viewer used? I made this on a Mac computer and checked using preview but it also seems to be ok if viewed with a acroread !?
The derivation of R= 2 L/tau.
I guess this can be found in a number of books. For me the fastes way was to sove the differential equation for the RL parallel C circuit using Maple.
the equations: i(t) = diff(u(t), t)*C and u(t) = -diff(i(t), t)*L - i(t)*R yield solutions containig the time dependent factor:
exp[(-R/(2*L) + sqrt(C^2*R^2 - 4*C*L)/(2*C*L))*t]
in the underdamped case the square root is imaginary and yields the oscillation, the dampinng tau is in the first part
-R/(2*L)*t => t/tau with tau= 2L/R.
Michael Monkenbusch